Step 8:
Calculate the distance from the imaged object (
X,Y, Z
)
and spacecraft position (
X
0
,
Y
0
,
Z
0
).
Step 9:
Calculate the value of
ψ
roll_atmos
and
ψ
pitch_atmos
. An ex-
ample of calculation (radian) as following:
(
)
ψ
θ
roll atmos
dem roll
R h
X X Y Y Z Z
_
=
+
−
(
)
+ −
(
)
+ −
(
)
•
∆
0
2
0
2
0
2
(26)
where,
R
is the mean Earth radius;
h
dem
is the object’s terrain
elevation interpolated from
SRTM
90 m digital elevation data.
Step 10:
Use Equation 25 to calculate the atmospheric refrac-
tion geolocation error corrected
ECEF
coordinate.
In the DMC3/TripleSat Constellation in-orbit commissioning
and early operation phase, the studied method was used to di-
rect georeferencing the DMC3/TripleSat 1 m resolution images.
Table 5 lists four sample DMC3/TripleSat Constellation images
with atmospheric refraction geolocation error correction. Since
the spacecraft off-pointing limit in the early phase, the four
sample images were all imaged in roll off-pointing mode. In
the future, we will investigate in the atmospheric refraction
geolocation error in both roll and pitch off-pointing mode.
Conclusions
As the propagation direction of
LOS
from satellite payload
in-orbit is deviated because of the atmospheric refraction, the
points of the detector, projection center and ground object are
no longer in a line. This is one reason why there are geoloca-
tion errors in the collinear rigorous geometric model estima-
tion. The paper describes how to use the
LOS
tracking geom-
etry algorithm,
ISO
standard atmospheric model, and Owens
atmospheric refraction index calculation method to estimate
the atmospheric refraction geolocation error and how to cor-
rect this error in the collinear rigorous geometric model.
The proposed method is especially suitable for the high
resolution agile optical satellite as images taken from a large
off-pointing view angle is more susceptible to the atmospher-
ic refraction. We also have applied the proposed method in
direct georeferencing DMC3/TripleSat Constellation 1 m reso-
lution images to improve the geolocation accuracy without
ground points.
However, we have to admit that atmospheric refraction is
one element that has made collinear geometric model non-
rigorous. Other elements, such as the light aberration and the
transmission delay of
LOS
, can also introduce geometric errors
in the collinear geometric model. Only when all the causes
that lead to inaccurate estimation of the interior orientation
and exterior orientation parameters are calibrated, can we
obtain a real accurate collinear rigorous geometric model.
Acknowledgments
This work was partly supported by the National High Tech-
nology Research and Development Program of China (No.
2013AA12A303) and Major Project of High Resolution Earth
Observation System (Civilian Part) (No. 65-Y40B01-9001-
13/15). The authors also thank the anonymous reviewers for
their construction comments and suggestions.
References
Bosen, J., 1960. A formula for approximation of the saturation vapor
pressure over water,
Monthly Weather Review
, pp. 275–276.
Crespi, M., F. Fratarcangeli, F. Giannone, and F. Pieralice, 2007.
SISAR: A rigorous orientation model for synchronous and
asynchronous pushbroom sensors imagery,
The International
Archives of the Photogrammetry, Remote Sensing and Spatial
Information Sciences
, XXXVI-1/W51, unpaginated CD-ROM.
Dowman, I., K. Jacobsen, G. Konecny, and R. Sandau, 2012.
High
Resolution Optical Satellite Imagery
, Whittles Publishing, 129 p.
Fan, D.Z., C.B. Liu, and T. Wang, 2011. Building and Validation
of rigorous geometric model of ALOS PRISM imagery,
Acta
Geodaetica et Cartographica Sinica
, 40(5):569–574.
Greslou, D., F. Lussy, and J. Montel, 2008. Light Aberration Effect
in HR Geometric Model,
The International Archives of the
Photogrammetry, Remote Sensing and Spatial Information
Sciences
, XXXVII-B1:859–864.
Gruen, A., and S. Kocaman, 2008.
Optical Sensors High Resolution:
Geometry Validation Methodology
, Technical report submitted to
ESA/ESRIN, 34 p.
Gyer, M., 1996. Methods for computing photogrammetric
refraction corrections for vertical and oblique photographs,
Photogrammetric Engineering & Remote Sensing
,
62(3):301–310.
Habib, A., S.W. Shin, K. Kim, C. Kim, K.I. Bang, E.M. Kim,
and D.C. Lee, 2007. Comprehensive analysis of sensor
modeling alternatives for high resolution imaging satellites,
Photogrammetric Engineering & Remote Sensing
, 73(11):1241–
1251.
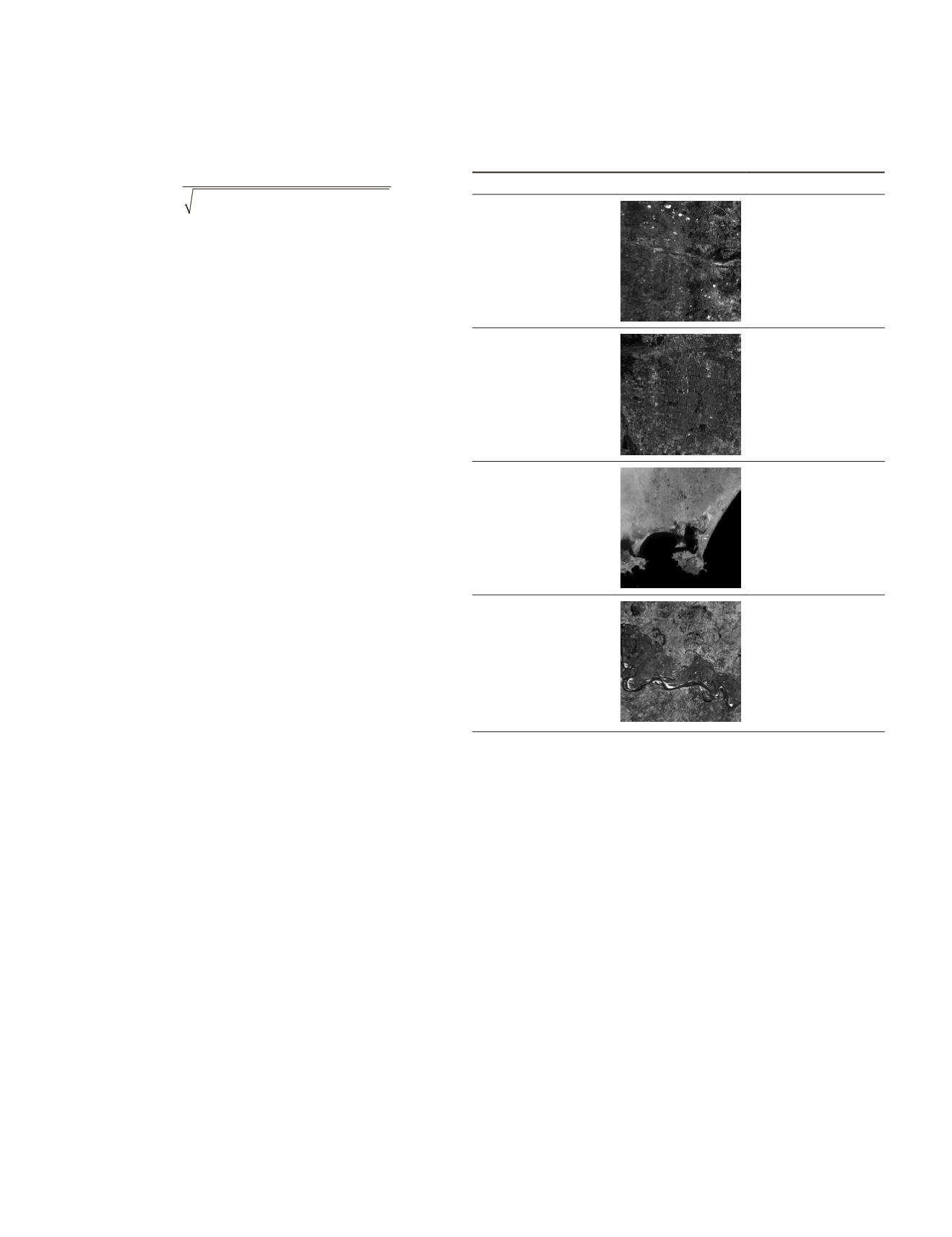
T
able
5. E
xperiment
R
esults
of
F
our
samples
DMC3/T
riple
S
at
C
onstellation
I
mages with
the
A
tmospheric
R
efraction
G
eolocation
E
rror
C
orrection
. T
he
value
of
X_
corr
is
the
A
tmospheric
R
efraction
G
eolocation
E
rror
C
orresponding
to
the
P
itch
O
ff
-
pointing
V
iew
,
and
the
V
alue
of
Y_
corr
is
the
A
tmospheric
R
efraction
G
eolocation
E
rror
C
orresponding
to
the
R
oll
O
ff
-
pointing
V
iew
.
Data
Browse Image
Geolocation Error (m):
Date:
23 Sep, 2015
Image ID:
D2000093VI
Location:
Guildford, UK
Off-pointing (degree):
Roll: -5.908
Pitch: 0
X_corr: 0
Y_corr: 0.31
Date:
2 Oct, 2015
Image ID:
D20000A3VI
Location:
Beijing, China
Off-pointing (degree):
Roll: -16.967
Pitch: 0
X_corr: 0
Y_corr: 0.82
Date:
13 Oct, 2015
Image ID:
D20000BEVI
Location:
Aden, Yemen
Off-pointing (degree):
Roll: 23.996
Pitch: 0
X_corr: 0
Y_corr: -1.54
Date:
16 Oct, 2015
Image ID:
D10000B1VI
Location:
Zhaoyuan,
Haerbin, China
Off-pointing (degree):
Roll: 0.001
Pitch: 0
X_corr: 0
Y_corr: 0
434
June 2016
PHOTOGRAMMETRIC ENGINEERING & REMOTE SENSING


