September 2019 Full - page 654

pixels, respectively, all less than 1 pixel. In the CCD direction,
the mean values of the forward, nadir, and backward views
are 1.266, 2.343, and 1.988 pixels, respectively, and the RMSE
values are 0.604, 1.468, and 0.999 pixels, respectively, which
are less than or close to 1 pixel. The viewing angle curves ob-
tained from Data A and Data B are very stable and have only
minor differences.
Comparing Data B with Data D, in the flight direction, the
mean values of the viewing angle curve differences are 2.852,
0.758, and 7.357 pixels for the forward, nadir and backward
views, respectively, and the RMSE values are 1.283, 0.542,
and 0.942 pixels, respectively, less than or close to 1 pixel. In
the CCD direction, the mean values of the forward, nadir, and
backward views are 3.998, 2.343, and 5.275 pixels, respective-
ly, and the RMSE values are 1.283, 1.466, and 3.862 pixels,
respectively. The differences between Data B and Data D are
significantly larger than the differences between Data A and
Data B, especially for the backward view CCD line array.
Four factors are responsible for this phenomenon:
1. For Data A and Data B, the acquisition time and location
are basically the same, and the acquisition time interval
between Data D and Data A andB is up to 5 months. The
flight area is different, and the imaging environment and
conditions also vary.
2. For Data D, the height altitude is 2600 m, which reduces
the ground resolution.
3. For Data D, 200 GCPs are collected postflight by puncture
means and field measurements. Because GCP selection is
greatly limited in urban areas, some GCPs are located on
housing roofs, flower beds, pool corners, and other places
with abrupt height variation. The GCPs’ accuracy for Data D
is lower than that for Data A and Data B.
4. For Data D, the imaging quality of the backward view CCD
is degraded for partial shutter occlusion, and this degrada-
tion also affects the point puncture accuracy.
Among the above four factors, factors 3 and 4 mainly
compensate for the large differences between Data B and Data
D shown in Table 6.
We calculate the final calibrated CAM files for the forward,
nadir and backward view CCD line arrays by averaging CAM
files from Data A, Data B, and Data D. The accuracy and
reliability of calibrated CAM files will be further validated in
uncontrolled DG experiments. Using the average CAM files, DG
was performed once again to assess the effect of the CAM files.
The DG results are shown in Table 7.
Table 7 shows that the calibrated CAM files can effectively
eliminate the positioning error caused by lens distortion, CCD
rotation, scaling, and other geometric deformation and signifi-
cantly improve the height positioning accuracy. However, the
improvement in planar accuracy is not as obvious.
Redirect Geopositioning Based on GNSS Lever Arms and IMU Boresight
Misalignment Calibration Values and CAM Files
Using both the average CAM files and updated GNSS/IMU
observations, DG was performed another time. The test results
are shown in Table 8. Table 8 shows that the positioning ac-
curacy in the X , Y, and Z directions is significantly improved
with the updated GNSS/IMU observations and CAM files and is
close to the accuracy level after AT and calibration. This find-
ing proves that the average calibration values of GNSS lever
arms and IMU boresight misalignment and CAM files are stable
and have good applicability.
For a more visual analysis, the experimental results for
Data B and Data C are plotted. The experimental results for
Data B in Tables 2, 3, 7, and 8 are plotted in Figures 13–16.
Figure 13 shows the residual error distribution of 108 control
points after DG. The left plot shows the residual distribution in
the plane (X and Y directions), and the right figure shows the
residual distribution in the height direction. Figure 14 shows
the residual error distribution after AT & Calibration. Figure 15
shows the residual distribution of the direct positioning using
CAM files. Figure 16 shows the residual distribution of the
direct positioning using both updated GNSS/IMU observations
and CAM files. The coordinates shown from Figure 13 through
ureFigure 16 adopt the local coordinate system.
As shown in Figure 13, for DG with GNSS/IMU observations
and initial CAM files, there are obvious systematic errors. The
plane residuals of different strips have obvious directional-
ity, but the variation in height residuals remains consistent.
In Figure 14, the remaining errors are mainly causality errors
since the systematic positioning errors caused by the GNSS
lever arms, IMU boresight misalignment, lens distortion, and
CCD deformation are effectively eliminated after AT & Calibra-
tion. Using GNSS/IMU observations and calibrated CAM files for
the second direct DG , Figure 15 shows that this approach can
significantly improve height accuracy but has a minor effect
on planar accuracy. With updated GNSS/IMU values and cali-
brated CAM files, Figure 16 shows that both height accuracy
and planar accuracy are effectively improved. From Figures
13–16, we can obtain the following preliminary conclusions:
The GNSS lever arms and IMU boresight misalignment mainly
affect the plane positioning accuracy of the GFXJ camera; The
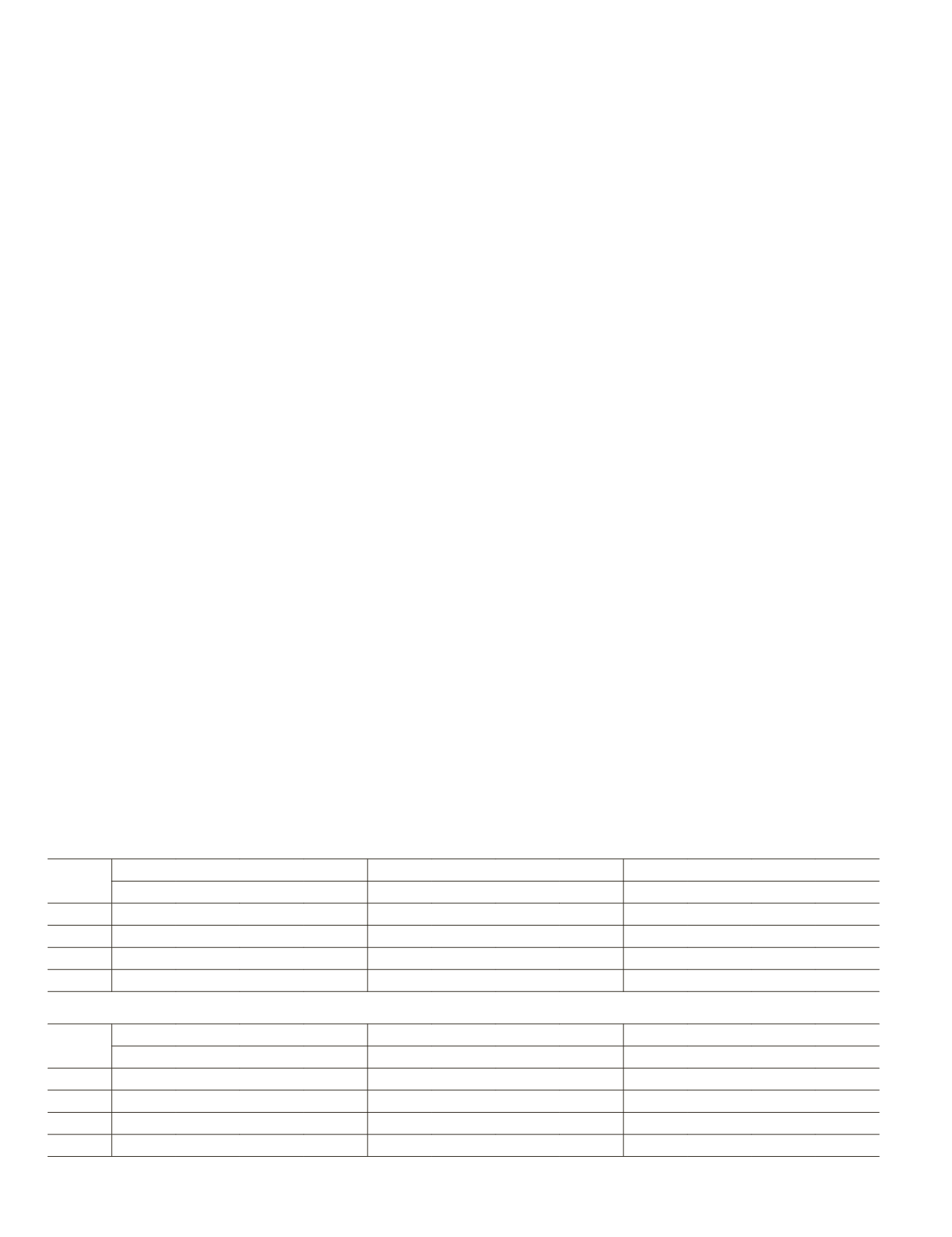
Table 7. Direct geopositioning based on CAM files.
Test
Data
Accuracy in X direction (meters) Accuracy in Y direction (meters) Accuracy in Z direction (meters)
Max Min Mean Std Max Min Mean Std Max Min Mean Std
Data A 4.763 −4.003 0.049 2.311 4.475 −3.833 1.264 3.211 1.188 −1.121 0.031 0.692
Data B 4.812 −4.142 0.722 3.543 4.363 −4.686 0.354 3.571 1.768 −0.798 0.560 0.823
Data C 4.681 −3.797 0.458 3.961 1.820 −2.196 −0.140 1.750 1.588 −1.599 0.454 0.779
Data D 6.501 −6.156 1.600 4.104 6.969 −6.231 1.138 3.152 3.259 −3.099 −0.200 0.908
Table 8. Direct geopositioning based on updated GNSS/IMU observations and CAM files.
Test
Data
Accuracy in X direction (meters) Accuracy in Y direction (meters) Accuracy in Z direction (meters)
Max Min Mean Std Max Min Mean Std Max Min Mean Std
Data A 0.561 −0.565 −0.002 0.235 0.641 −0.651 0.002 0.267 0.823 −0.878 −0.010 0.278
Data B 0.687 −0.290 −0.013 0.119 0.403 −0.210 0.024 0.110 0.492 −0.556 0.009 0.256
Data C 0.511 −0.182 −0.007 0.123 0.234 −0.472 0.006 0.158 0.703 −0.865 0.015 0.267
Data D 0.769 −1.024 −0.315 0.397 0.797 −0.835 0.011 0.313 1.082 −0.991 0.247 0.309
654 September 2019 PHOTOGRAMMETRIC ENGINEERING & REMOTE SENSING