relative localization accuracy is
not the lowest. In addition, the tie
points (Nos. 1, 4, 5, 6, 9, 10) are
nearly collinear. When those tie
points are used, the
BA±LS
algo-
rithm fails, and the other algo-
rithms can acquire the result with
certain accuracy.
In “C6-C7” and “C8-C9,” the
noise in the image generated by
the camera system will damage the
quality of the image. Hence, the tie
points have low extraction preci-
sion at the “C6” and “C8” stations,
resulting in low rover pose estima-
tion.
In (Wang,
et al.
, 2014; Wan,
et
al.
,2014), the experiment results
prove that
BA
provides more ac-
curate localization results (1 %~4
%) than dead-reckoning in the
relative distance (2m~7m). While
corresponding points are found in
restrict regions formed by initial
localization results from dead-reck-
oning, localization is refined by
BA
to gain accurate location results
(Wan,
et al.
,2014). Different from
the
BA
algorithms,
WTLS
provides
the relative localization results
(3.58%, 3.72%, 5.17%, 8.11%) in
the relative distance (6.7m, 6.5m,
5.4m, 4.8m). However,
WTLS
has
a high precision of relative local-
ization results (2.56%~8.3%) at a
greater range (7m~13.8m). Experi-
mental results show that the rover’s
pose estimation accuracy does
not decrease or increase with the
distance. The precision of image point extraction, the distri-
bution of the tie points and the distance between the vary-
ing stations are the main factors that affect the rover’s pose
estimation accuracy.
2. Efficiency
. The calculation efficiencies of
WTLS
and
TLS
are well above that of
BA
(parallaxBA,
BA
+
LM
, and
BA
+
LS
).
As a repeated process of resection and forward intersec-
tion to jointly estimate camera poses and 3D structure,
BA
requires many iterations. At the same time, the structure of
the normal equation matrix and observation vector is more
complex in the calculation process. To solve the problem
of the ill-conditioned normal equations, parallaxBA intro-
duces the damping factor into the diagonal elements of the
normal equation matrix, which is denoted by the
LM
algo-
rithm. However, the
LM
algorithm still requires the iterative
correction of the damping factor with the selected weight
iteration method. In sum, the calculation efficiency based
on
BA
(7 iterations) is drastically reduced. In contrast, the
WTLS
algorithm does not need multiple iterative calcula-
tion, and the structure of equations (15, 16) is relatively
simple, while the unknown parameters include only the
lunar rover poses. More importantly, the
WTLS
algorithm
avoids the appearance of the ill-conditioned normal equa-
tions in the
BA
model. To access the calculation efficiency,
the calculation times of
WTLS
and
BA
from the process of
inputting the matching image points to the calculation of
the lunar rover pose parameters are listed in Table 11.
3. Convergence
. In the actual test, the yaw angles
k
of the
lunar rover are very large, and the image network of the
tie points is sparse. Having considered that the observa-
tion errors exist in the coordinate system transformation
framework, the initial data of the camera poses will be
worse when the observation value of lunar rover poses
from the
IMU
has inadequate accuracy. In this case, a poor
initialization of camera poses can cause the divergence
of parallaxBA,
BA
+
LS
, and
BA
+
LM
and the singularity of
the normal equation matrix. That is, the initial values of
the poses are critical in
BA
. However, the accurate pose
parameters of the lunar rover can be obtained directly us-
ing the
WTLS
algorithm, and the initial data of rover poses
from the
IMU
is not necessary. When the Chang’E-3 lunar
rover works in orbit, because of the slip of the wheel, the
position estimation obtained by odometry is not the actual
resulting position of the rover. The pose estimation results
of China’s first lunar rover using parallaxBA and
WTLS
can
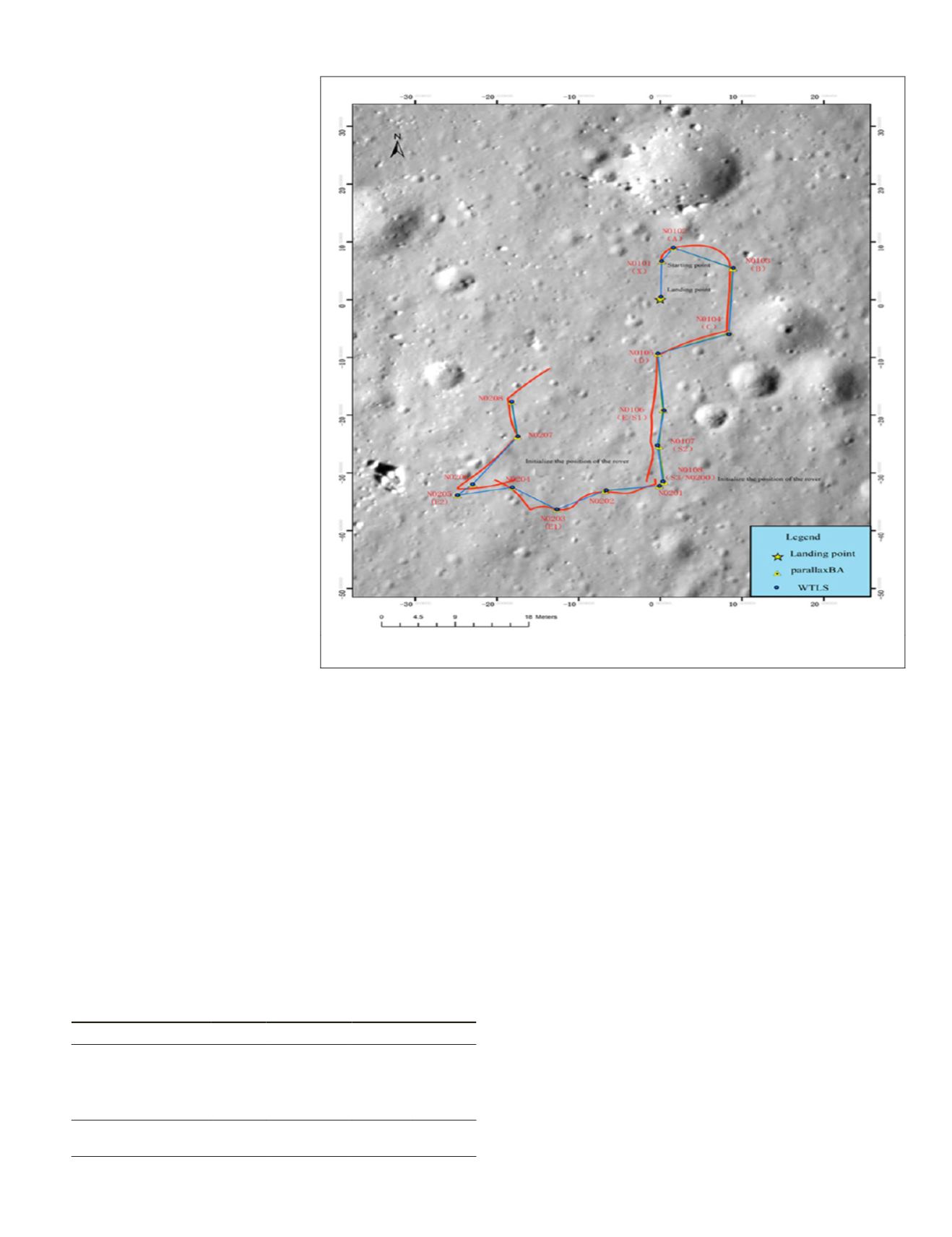
be seen in Figure 8.
Figure 8. The lunar rover’s pose estimation results using the parallaxBA (triangle) and
WTLS
(circle) methods. The red line represents the odometry data of the rover in real time.
Table 11. Calculation time of the different rover’s localization
methods (ms)
a)
.
Number of tie points WTLS parallaxBA BA+LS BA+LM
5
1
565
452
568
6
1
578
461
580
9
1
591
478
589
12
1
584
493
585
a) the test environment is on an Intel Core i5@2.50 GHz, 2 GB Win-
dows system
PHOTOGRAMMETRIC ENGINEERING & REMOTE SENSING
October 2018
615


