squares are from image set 2 in Table 2), the leftmost 3000
CCDs
were selected as primary
CCDs
, and the image from set
1 (red square in Figure 11) was selected as the benchmark
image for the self-calibration. Thus, the smaller primary
CCD
could provide enough absolute control for the paired stereo-
scopic images and its dependent calibration field could be re-
duced as far as possible. The dense
GCPs
(red points in Figure
11) that were distributed in a narrow strip across the primary
CCD
were automatically matched from the high-accuracy refer-
ence
DOM
and
DEM
of ZY3. Based on the
GCPs
, the installation
angles of the benchmark image and the internal parameters of
the primary
CCD
were calibrated using the traditional stepwise
calibration method. Then, based on the calibrated internal
parameters of the primary
CCD
, the installation angles of im-
age set 2 (blue square in Figure 11) were calibrated using
GCPs
(red points in Figure 11) extracted from the high-accuracy
reference data of ZY3. These results are shown in Tables 3
and 4. The calibrated
WFV3
and
WFV4
installation angles were
close to the design values shown in Figure 1. The small differ-
ence between the calibrated installation angles of the paired
stereoscopic images was likely caused by attitude and orbit
measurement error, and by the change in installation angles.
Based on the absolute geometric constraint of the primary
CCD
, the relative coordinate systems of paired stereoscopic im-
ages can be precisely determined and applied in subsequent
self-calibration using tie points and the reference
GDEM2
. The
dense tie points (blue points in Figure 11) in the paired ste-
reoscopic images were matched and distributed within a nar-
row strip around the
GCPs
used previously, and therefore the
tie points and
GCPs
had a similar imaging time in each image,
which ensured sufficient precision of the relative coordinate
systems. Using our proposed model, the internal parameters
of the whole
CCD
were calibrated. Figures 12a, 12b, 13a, and
13b show the
LOS
residual error of the tie points along and
across the track after calibration. Its random distribution
without systematic deviation indicates that internal distor-
tion was well compensated. Figures 12c and 13c show the
internal distortion curve of the whole
CCD
. The high-accuracy
reference data of ZY3 were used to evaluate the calibration
accuracy, and Figures 12d and 13d show the positioning
residual error of the check points after calibration. The direc-
tions of the plane error vectors were different and random,
demonstrating that the systematic errors of the
WFV3
and
WFV4
cameras were effectively eliminated by self-calibration.
The positioning error of both the
WFV3
and
WFV4
cameras
was decreased to less than 1.0 pixel after self-calibration (Ta-
ble 5), which fully validates the effectiveness of the proposed
method in terms of detecting and compensating for internal
distortion. In addition, its calibration accuracy was similar to
that of the traditional method totally based on a calibration
field (Jiang
et al.
2014; Wang
et al.
2014), indicating that the
self-calibration approach based on elevation residual error
has similar calibration ability to the traditional method.
The internal relative accuracy of check images was evalu-
ated using the relative positioning accuracy. We can calculate
the relative positioning accuracy after an affine transformation
of the check images. Exterior systematic positioning offset can
be eliminate by the following rational polynomial coefficients
(RPC) affine model based on a few
GCPs
(Hanley, Yamakawa
and Fraser 2002; Fraser and Hanley 2003):
x a a x a y
h
y b b x b y
h
x
y
+ + + =
(
)
+ + + =
(
)
0 1 2
0 1 2
RPC lat lon
RPC lat lon
,
,
,
,
.
(10)
We selected three groups of images covering three different
areas in China.
GCPs
of each image were automatically extract-
ed (by matching) from the reference
DOM
and
DEM
of ZY3. The
exterior systematic positioning offset was eliminated by some
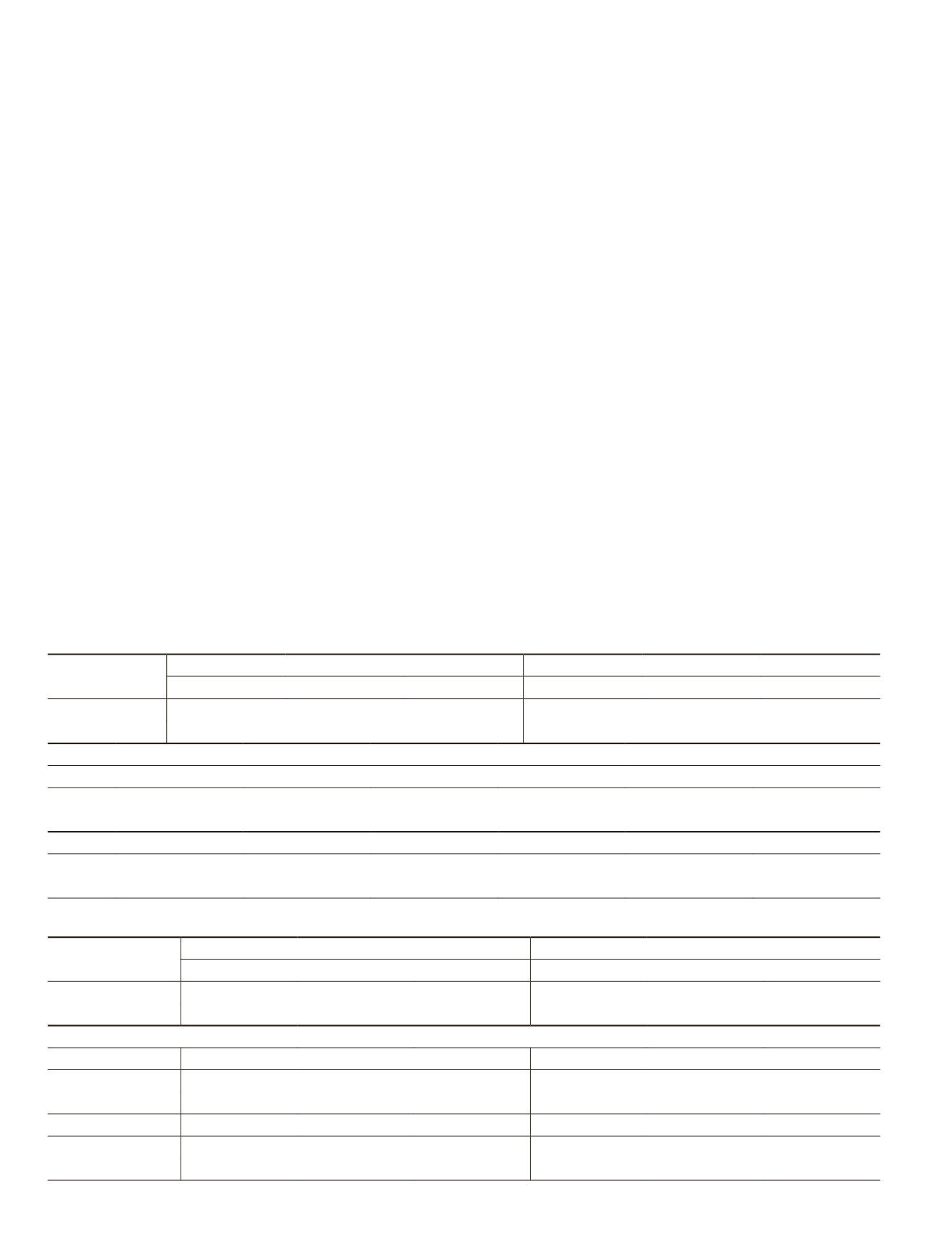
Table 3. Calibrated parameters of the primary charge-coupled device obtained from the
WFV3
camera.
Installation Angles
Image Set 1 (°)
Image Set 2 (°)
Roll
Pitch
Yaw
Roll
Pitch
Yaw
Initial
8.000 000
0.000 000
0.000 000
8.000 000
0.000 000
0.000 000
Calibrated
7.998 561
−0.049 282
0.006 435
a
0
a
1
a
4
a
5
Initial
0.000 000e−00
0.000 000e−00
0.000 000e−00
0.000 000e−00
0.000 000e−00
0.000 000e−00
Calibrated −3.435 100e−06
1.082 832e−08
−3.521 308e−11
3.004 587e−14
−1.039 845e−17
1.262 255e−21
b
0
b
1
b
2
b
3
b
4
b
5
Initial
1.444 585e−01
−2.832 520e−05
0.000 000e−00
0.000 000e−00
0.000 000e−00
0.000 000e−00
Calibrated 1.478 479e−01
−2.620 183e−05
5.148 207e−10
−4.316 973e−14
−8.867 116e−18
2.015 992e−21
Table 4. Calibrated parameters of the primary charge-coupled device obtained from the
WFV4
camera.
Installation angles
Image Set 1 (°)
Image Set 2 (°)
Roll
Pitch
Yaw
Roll
Pitch
Yaw
Initial
24.000 000
0.000 000
0.000 000
24.000 000
0.000 000
0.000 000
Calibrated
23.997 432
−0.065 298
0.006 284
24.000 695
−0.066 244
0.009 863
Internal Parameters
a
0
a
1
a
2
a
3
a
4
a
5
Initial
0.000 000e−00 0.000 000e−00 0.000 000e−00 0.000 000e−00 0.000 000e−00 0.000 000e−00
Calibrated
−4.679 481e−05 1.450 910e−08 −1.672 357e−11 9.339 181e−15 −2.116 493e−18 1.495 340e−22
b
0
b
1
b
2
b
3
b
4
b
5
Initial
1.444 585e−01 −2.832 520e−05 0.000 000e−00 0.000 000e−00 0.000 000e−00 0.000 000e−00
Calibrated
1.479 127e−01 −2.621 496e−05 5.714 532e−10 −1.146 885e−13 2.262 074e−17 −2.573 790e−21
822
November 2019
PHOTOGRAMMETRIC ENGINEERING & REMOTE SENSING


